The branch of mathematics that deals with the study of the analysis, collection, presentation, interpretation, and organization of data is known as Statistics. We can say that it is a mathematical discipline to collect, summarize data. As far as the JEE exam is concerned, statistics is an important topic. Students can expect 1-2 questions from this topic.
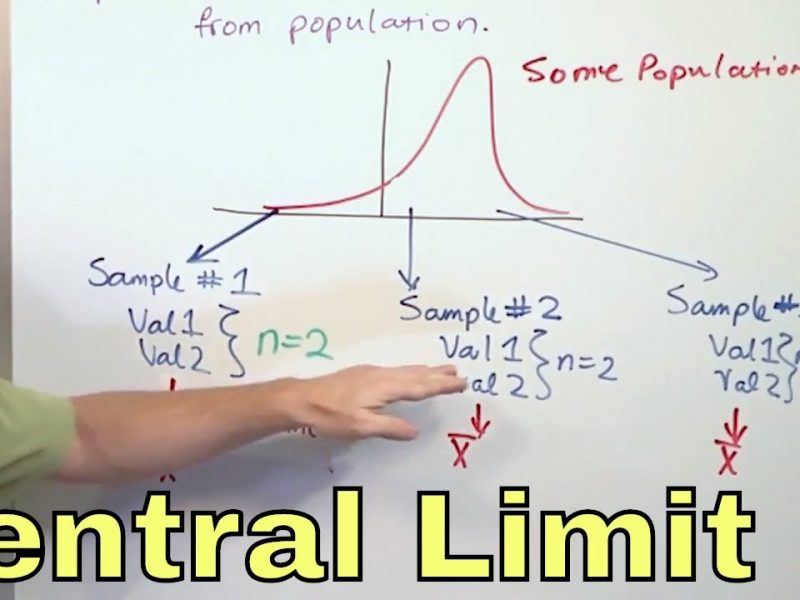
The basics of statistics comprise the measure of central tendency and the measure of dispersion. The central tendencies include mean, median and mode and dispersions consist of variance and standard deviation. The Central Limit Theorem (CLT) is an important topic in statistics. We can apply CLT to all types of probability distributions. Exceptions are also there. According to this theorem, for any population with mean and standard deviation, the distribution of the sample mean for sample size N has mean μ and standard deviation σ / √n .
Central Limit Theorem Statement
This theorem states that whenever a random sample of size n is taken from any distribution with mean and variance, then the sample mean will be approximately normally distributed with mean and variance. The higher the value of the sample size, the better the approximation to the normal. Following are some assumptions of CLT.
- Size of the sample must be sufficiently large.
- Samples should be drawn randomly following the condition of randomization.
- Samples should not influence the other samples.
- Samples drawn should not be dependent on each other.
Formula Of CLT
The formula is given as “Z = (x – – μ)/(σ /√n)”. Here Z is any random variable, μ is the mean, σ is the standard deviation and n is the sample size.
Properties of CLT
Following are some properties of CLT.
- Sampling is a form of any distribution with mean and standard deviation.
- When the sampling distribution is normal, the sampling distribution of the sample means is an exact normal distribution for any sample size.
- If the value of n is large, the sampling distribution of the sample mean will be approximately normally distributed with a mean and a standard deviation is equal to σ/√n.
Applications Of CLT
Some applications of CLT are given below.
- This theorem is used in finding the mean family income in a particular country.
- Observing the sample distribution, this theorem can tell whether the sample belongs to a particular population.
- If we increase the samples taken from the population, the sample mean deviation decreases. This helps in calculating the mean of the population in a more accurate manner.
There is another important concept in statistics which helps in establishing a relation between predicted and actual values obtained in a statistical experiment. This is called the Correlation Coefficient. The calculated value will explain the exactness between the predicted and actual values. The value will lie between -1 and +1. If the value is negative, then it shows dissimilarity between the two variables. A positive value will indicate a similar and identical relation between the two variables.
The Pearson’s coefficient is denoted by ρ (rho). It is the covariance of two variables divided by the product of their standard deviations. ρ can be expressed in terms of mean and expectation also.











Comments